We tackle a wide variety of problems at the forefront of interdisciplinary applied mathematics and engineering science fundamentals. We focus on complex, multiphase systems, using cutting-edge analytical and computational tools to develop a generic fundamental understanding of systems for which there is currently no existing theory.
Each of these projects is overseen by Professor Serafim Kalliadasis. Listed below are other current and former group members and collaborators with expertise in these areas. These projects are currently ongoing and are funded by a variety of EPSRC, ERC and EU grants. Our publications page contains details of our contributions. You can also read about some of our recent research highlights.
| Title | Collaborators - contributors |
|---|---|
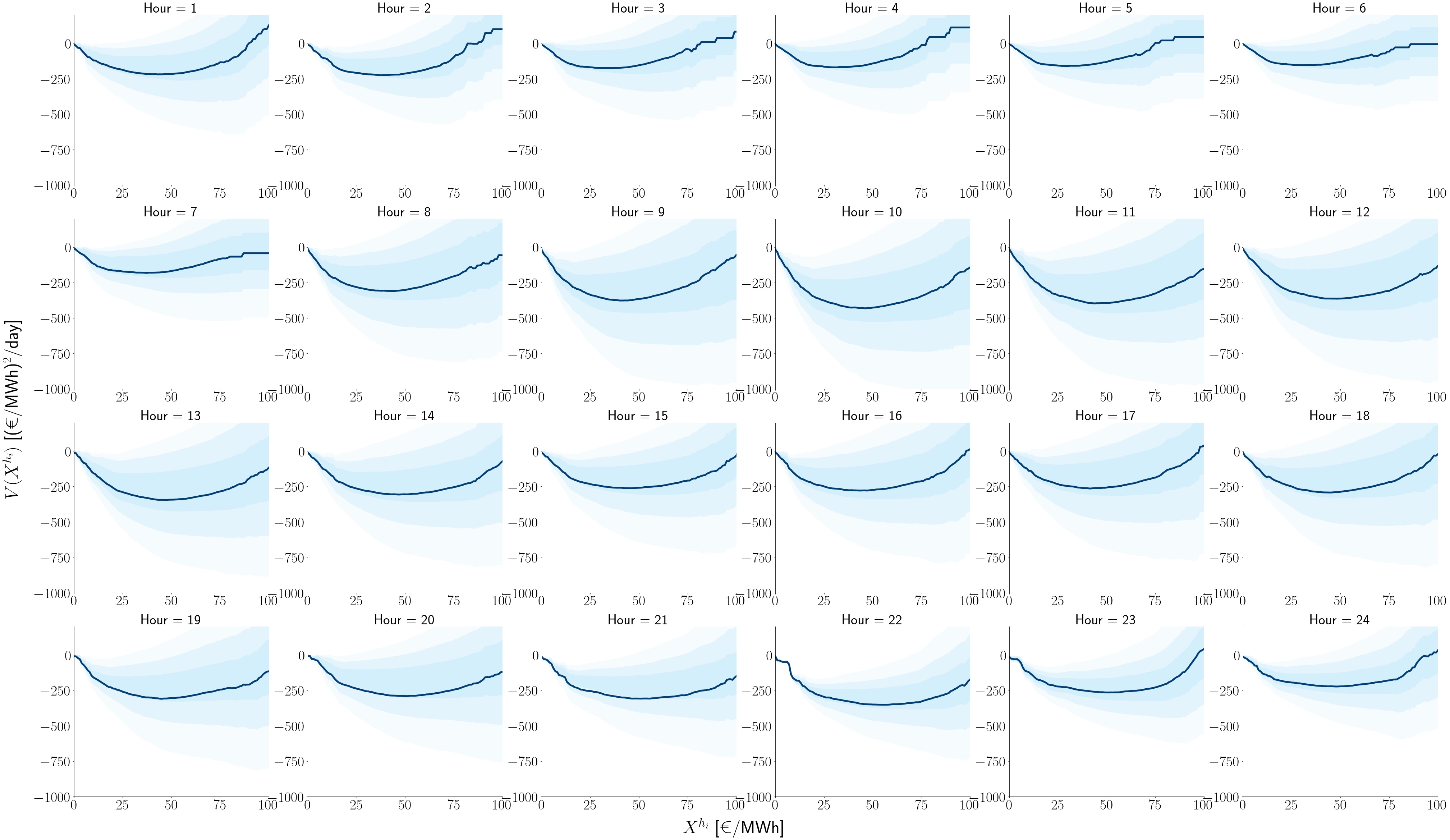
| Data-driven modelling of the electricity day-ahead markets | Mr Antonio Malpica-Morales, Dr Miguel A. Durán-Olivencia |
| Physics-informed Bayesian inference of external potentials in classical density functional theory | Mr Antonio Malpica-Morales, Dr Peter Yatsyshin, Dr Miguel A. Durán-Olivencia |
| Surface nanodrops and nanobubbles: a classical density functional theory study | Dr Peter Yatsyshin |
| Unconditional bound-preserving and energy-dissipating finite-volume schemes for the Cahn-Hilliard equation | Dr Rafael Bailo, Prof José A. Carrillo, Dr Sergio P. Perez |
| Please use the menu below to see some results from our recent projects | |
Summaries of selected projects
- Data-driven modelling of the electricity day-ahead markets
- Physics-informed Bayesian inference of external potentials in classical density functional theory
- Surface nanodrops and nanobubbles: a classical density functional theory study
- Unconditional bound-preserving and energy-dissipating finite-volume schemes for the Cahn-Hilliard equation
Data-driven modelling of the electricity day-ahead markets
Physics-informed Bayesian inference of external potentials in classical density functional theory
Surface nanodrops and nanobubbles: a classical density functional theory study
Unconditional bound-preserving and energy-dissipating finite-volume schemes for the Cahn-Hilliard equation