A key feature of quantum mechanics is that systems can be in a superposition of their basis states. These coherent superpositions are responsible for many of the counter-intuitive predictions of quantum mechanics, such as in a double slit experiment where an interference pattern is seen due to each single particle being in a superposition of passing through each individual slit.
The ability to manipulate superpositions of quantum states underpins emerging quantum technologies such as quantum computing, and more generally studying the interference between states is of importance in investigations into the fundamentals of quantum mechanics.
The ability for a quantum particle to be in a coherent superposition of physical states is an important distinction between classical and quantum mechanics, and efforts to properly define and quantify this coherence are relatively recent [1, 2]. Determining if a quantum system truly is in a coherent superposition of states or if it is simply in a classical probabilistic mixture can be difficult, since such properties must be determined statistically by repeated measurements.
We can define a pure quantum state using the following formula,

where our quantum system is defined in terms of the basis states {|j>}, and is said to be k-coherent is there are at least k nonzero coefficients ζj.
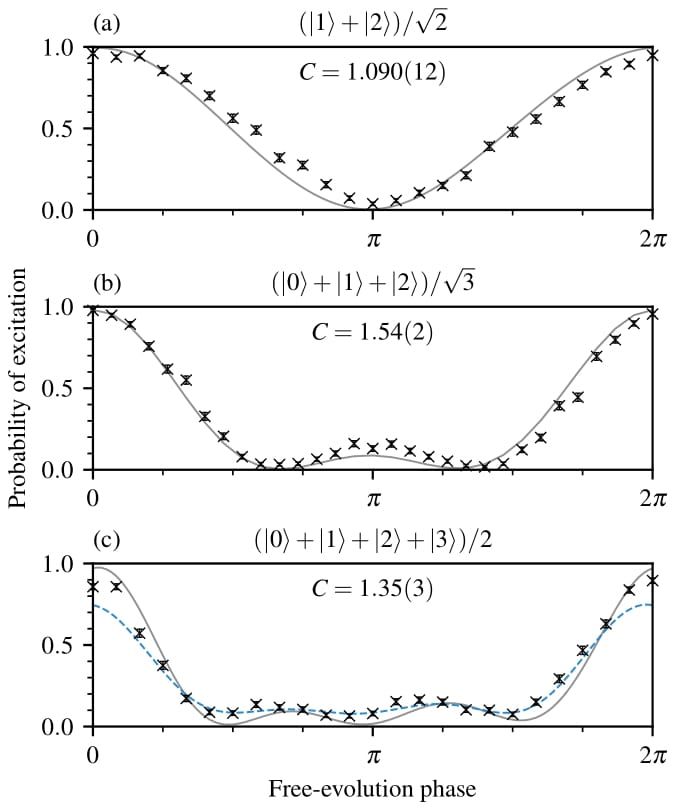
The objective of our research is to define a coherence metric with single number that can be measured using a simple experiment. The certifier used in our experiments is a function of normalised moments given by the expression,

For a probability distribution, the first moment is the expected value, with the third moment being the skewness of the distribution. Our certifier takes the ratio between these values[3],

which is able to overcome any measurement imperfection to prevent any over-estimates in the number of coherently superposed amplitudes[4]. Obtaining a value of C greater than 1 requires 2-coherence, greater than 5/4=1.25 requires 3-coherence, and greater than 179/96≈1.86 is necessary to certify 4-coherence in a Hilbert space of arbitrary dimention [3].
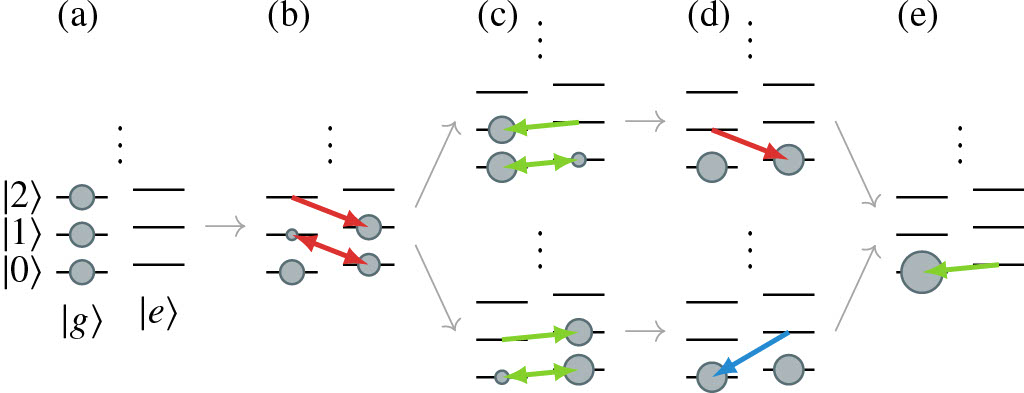
The motional states of a trapped ion provide the ideal system to demonstrate this certifier as each physical quanta of motion in the system is an oscillator Fock state . Without the ability to directly probe these states, we instead project the motional state onto the optical qubit of our ion using an interference pattern method. It is this probability distribution in which we apply our certifier .



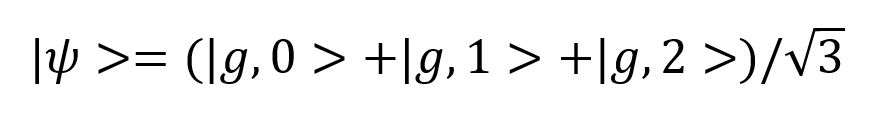
.jpg)